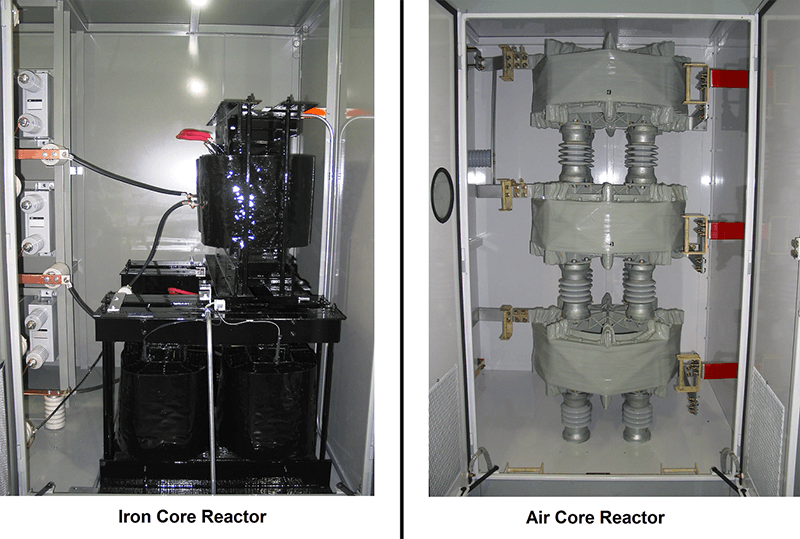
Summary: Controllix Corporation transitioned a coastal utility from using open rack style capacitor banks to their advanced metal-enclosed capacitor banks. This change notably improve...
Summary: Controllix Corporation transitioned a coastal utility from using open rack style capacitor banks to their advanced metal-enclosed capacitor banks. This change notably improve...Guide for Plant Engineers
Power Factor and Harmonics
Power factor is defined as real power, the power that does work, divided by apparent power, the power that must be delivered in order to get the work done. Real power is measured in kilowatts (kW) and apparent power is measured in kilovolt amps (kVA.) Power factor is sometimes denoted as “PF.” Mathematically, PF = kW/kVA. Values of PF range from unity (1), where all the power delivered does useful work, to zero (0), where no useful work is done for the power delivered.
Another way to state this definition is to say that PF is a measure of the efficiency of utilization of a power distribution system. The closer PF is to unity, the more of the electrical system capacity is being used to do useful work.
With linear loads, PF depends on the phase relationship between the current sine wave and the voltage sine wave. When these two waves are in phase, PF is unity and there is no system capacity wasted.
Linear loads such as resistance heaters and incandescent lights are 100% efficient in converting real power into heat and have a PF of unity.
Induction motors, in addition to real power, require reactive power, measured in kilovolt amps reactive (kVAR). The reactive current that flows in the system creates a magnetic field that enables the motor to operate, but does not contribute to the work done by the motor.
Reactive current also causes the current wave to lag the voltage wave, a process called displacement.
The apparent power (kVA) for a motor is equal to the square root of the sum of kW2 plus kVAR2. Since the apparent power for a motor is larger than the active power, PF is less than unity.
The PF for a system powering only linear loads is called the “displacement power factor” (the “DPF” on your meter). Unless the loads are pure resistance, this PF will be less than unity.
Non-linear loads draw harmonic currents, which are similar to reactive currents in that they do no real work but use up system capacity. The harmonic currents are often represented by the term “harmonic reactive power,” or kVAH. The apparent power for a non-linear load is equal to the square root of the sum of kW2 plus kVAR2 plus kVAH2. The presence of harmonics increases the apparent power that must be delivered to do a certain amount of work, and therefore lowers PF. The PF term often used to describe a system with both linear and non-linear loads is “true power factor,” or simply “power factor” (the “PF” on your meter).
If harmonic currents are introduced into a system, the true power factor is always lower than the displacement power factor. For example, the displacement power factor for a computer is close to unity, usually about 0.95, whereas the true power factor, which includes harmonics, is around 0.7. For both linear and non-linear loads, the result of extra current that does no real work, whether reactive current or harmonic current, is a reduced capability for the system to support useful loads. In effect, much of the transformer, wiring, and circuit breaker capacity is wasted carrying reactive or harmonic currents.
Many utilities make the customer pay for wasted system capacity by charging a “power factor penalty,” which is based on the numerical power factor. The further this number deviates from unity (the more system capacity being wasted), the larger the penalty.
In the past, most utilities measured only the displacement PF in determining power factor charges. Thus customers who had large numbers of non-linear loads were not paying the cost of the extra capacity needed to deliver harmonic currents. With the advent of effective electronic utility meters that can measure true power factor, some utilities are now charging customers penalties based on this value.
If your utility is measuring true power factor and charging you accordingly, it could benefit you to investigate harmonic mitigation to increase your power factor and reduce the penalties.